Dimensional Formula of Power

The dimensional formula of Power is

[ M¹  L²  T^-3 ]

where [M], [L], and [T] are the fundamental quantities: Mass, Length, and Time.

From the above formula, we can derive the dimensions of power as (1, 2, -3).

---

How to calculate the dimensional formula of power?

For this derivation, we first need to know some of the formulas:

Quantity	Formula
Power (P)	Work (W) / Time (T)
Work (W)	Force (F) * Displacement (D)
Force (F)	Mass (m) * Acceleration (a)
Acceleration (a)	Velocity (v) / Time (T)
Velocity (v)	Displacement (D) / Time (T)

Now, let’s use these formulas to find the dimension of power.

Derivation

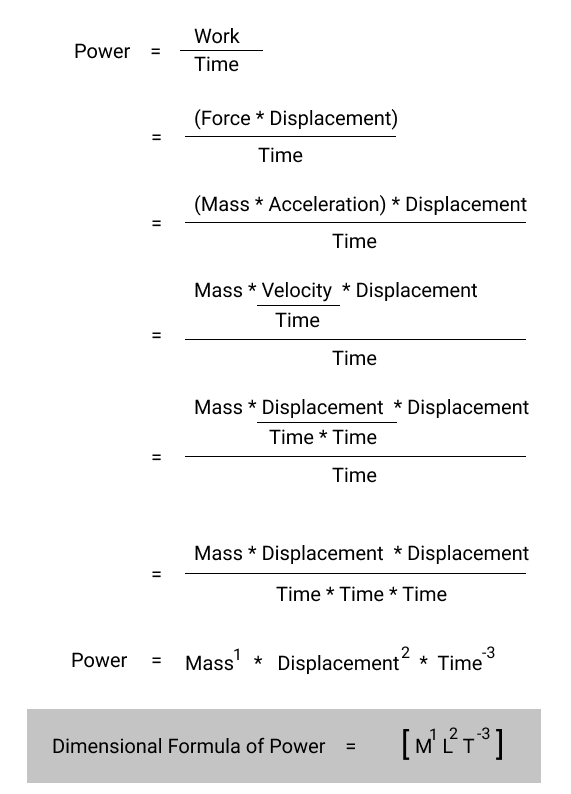
Power = Work / Time

      = (Force * Displacement) / Time  {using formula of Work}

      = (Mass * Acceleration * Displacement) / Time  {using formula of Force}

      = (Mass * (Velocity / Time) * Displacement / Time  {using formula of Acceleration}

      = (Mass * (Displacement / (Time * Time) ) * Displacement ) / Time  {using the formula of Velocity}

      = (Mass * Displacement * Displacement ) / (Time * Time * Time)

      = (Mass * Displacement2 ) / Time3

Power = Mass * Displacement2 * Time-3

Now, let’s convert this formula in terms of fundamental quantity [M] (Mass), [L] (Length), and [T] (Time)

• Mass is represented in terms of [M]
• Displacement is represented in terms of [L]
• Time is represented in terms of [T]

Hence, 

Dimensional Formula of Power = [M1 L2 T-3]

From the formula, the dimensions are

• 1 for Mass
• 2 for Length
• -3 for Time

If you want to learn more about dimensional analysis, visit Dimensional Formula and Equations of Physical Quantity.