Dimensional Formula of Energy

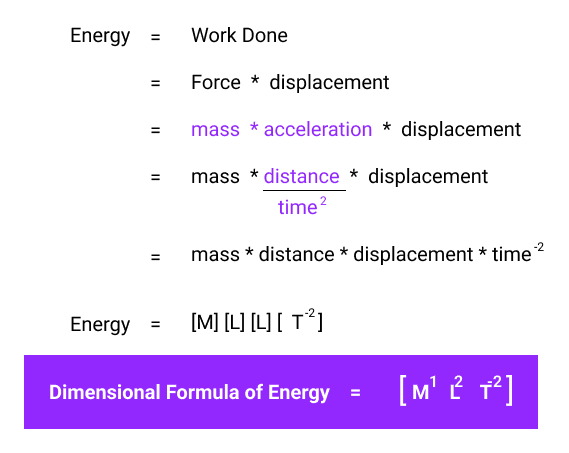
The dimensional formula of Energy is 

[ M1  L2  T-2 ]

Here, [M], [L], and [T] are the fundamental quantities: Mass, Length, and Time.

From the above formula, it’s clear that the dimension of energy is 1, 2, -2.

How to calculate the dimensional formula of energy?

We can derive this formula using the definition of energy.

In physics, we can define energy as the ability to do work. Hence, mathematically,


Energy = Work Done

We know that work is equal to force multiplied by displacement.


  Work Done = Force * displacement

Hence, our formula becomes


  Energy = Force * displacement

Also, Force = mass * acceleration. Hence,


  Energy = mass * acceleration * displacement

We know 

  • acceleration = speed / time
  • speed = distance / time
  • acceleration = distance / time2

Therefore,


  Energy = (mass * distance * displacement ) / time2

  Energy = (mass * distance * displacement * time-2 )

Dimensionally, we use

  • [M] to represent mass
  • [L] for both distance and displacement
  • [T] to represent time

Hence, the dimensional equation of energy becomes [ M L2 T-2 ].

Numerical Derivation of dimensional formula of energy

Frequently Asked Questions

1. What is the dimensional formula of Kinetic Energy?

The dimensional equation of Kinetic Energy is:

[ M L2 T-2 ]

Derivation

We know the formula of Kinetic Energy (KE):


  KE = (1 / 2 ) mass * velocity2

Here, velocity = displacement / time.


  KE = (1 / 2) mass * ( displacement / time )2

  KE = (1 / 2) mass * displacement2 * time-2

Hence, dimensionally,

Kinetic Energy = [ M1 L2 T-2 ]

2. What is the dimensional formula of Potential Energy?

The dimensional equation of Kinetic Energy is:

[ M L2 T-2 ] 

Derivation

We know the formula of Potential Energy (PE):


  PE = mass * gravitational field * height

In terms of units, we can deduce

  • mass into kilogram
  • the gravitational field into meter / second2
  • height into meter

So,


  PE = kilogram * (meter / second2) * meter

  PE = kilogram * meter2 * second-2 

And, dimensionally, we represent

  • kilogram as [M]
  • meter as [L]
  • second as [T]

Hence, dimensionally, we can represent Potential Energy as [ M1 L2 T-2 ].

Related Articles: