Dimensional Formula of Force

The dimensional formula of force is 

[ M1 L1 T-2 ]

where, [M], [L], and [T] represent fundamental quantity Mass, Length, and Time.

Now, let’s see how we can derive this formula.

How to calculate the dimension formula of force?

For this derivation, we need to revise some of the basic physics formulas.

QuantityFormula
Force (F)Mass (m) * Acceleration (a)
Acceleration (a) Velocity (v) / Time (T)
Velocity (v)Displacement (D) / Time (T)

Now, let’s use these formulas to find the dimension of the force.


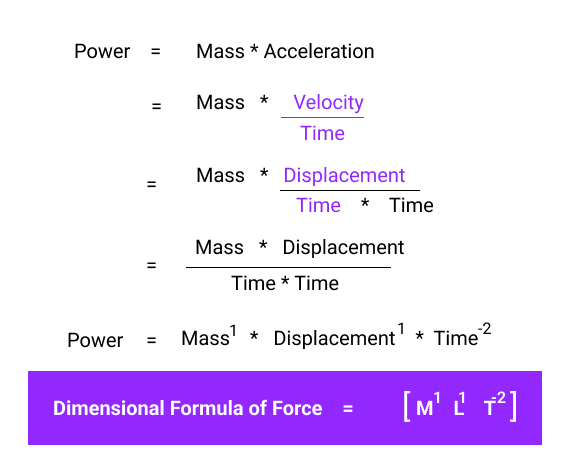
Force    =   Mass * Acceleration

         =   Mass * (Velocity / Time)     [using formula of acceleration]

         =   Mass * (Displacement / (Time * Time) )   [using formula of velocity]

         =   ( Mass * Displacement ) / Time2

         =   Mass1 * Displacement1 * Time-2

Dimensionally, we use

  • [M] to represent mass,
  • [L] for displacement
  • and [T] for time

Now the above formula becomes


  Force = [ M1] * [L1] * [T-2 ]

Force = [ M1] * [L1] * [T-2 ]

Hence, the dimensional formula of Force is [ M1  L1  T-2 ].

The dimensions of force is 1, 1, -2 in terms of Mass, Length and Time.

Frequently Asked Questions

Related Articles