Radius of Gyration

In this article, we will learn about the radius of gyration in physics and its applications. We will also calculate its value for a thin rod and solid sphere.

First, let’s start with the definition.

Radius of Gyration is an imaginary distance from the axis of rotation to a point where the total mass of the body is concentrated so that the moment of inertia about the axis remains the same.

It basically relates the moment of inertia about the particular axis to the area of rotation.

---

What is the formula of a radius of gyration?

Suppose we have a body of mass M, then the moment of inertia is

  Inertia (I) = MK2

Here, K denotes the radius of gyration.

From the above equation, we can derive the formula of the radius of gyration as

  K = √(I / M)

So, K is the square root of the I (moment of inertia) divided by M, the total mass of the body.

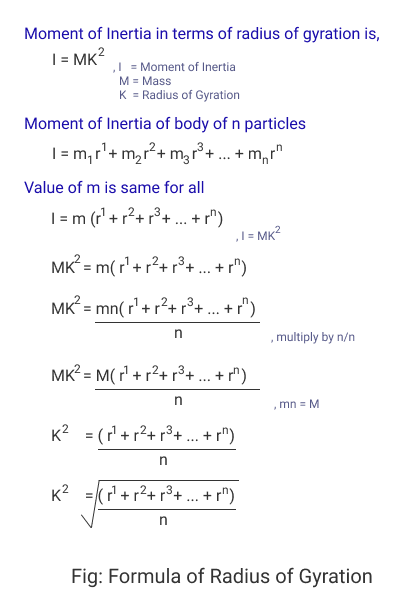
Now, suppose the body consists of n particles each of mass m and r₁, r₂, r₃, …., r_n be the perpendicular distances of particles from the axis of rotation.

Then, the moment of inertia of the body about the axis of rotation is

  I = m1 r12 + m2 r22 + m3 r32 + …… + mn rn2

As per our assumption, each particle is of the same mass, m. So, our equation becomes

  I    = m (r12 + r22 + r32 + …… + rn2)

Multiplying the right-hand side by n / n, we get

  I    = mn (r12 + r22 + r32 + …… + rn2) / n

We know the total mass of the body, M = mn.

  I    = M (r12 + r22 + r32 + …… + rn2) / n

From the earlier equation, we have I = MK². So,

  MK2 = M (r12 + r22 + r32 + …… + rn2) / n
  
  canceling M on both sides

  K2 = (r12 + r22 + r32 + …… + rn2) / n

  K = √( (r12 + r22 + r32 + …… + rn2) / n)

  K = root square mean distance of particles from the axis of rotation.

Hence, we can also define the radius of gyration as the root-mean-square distance of the various particles of the body from the axis of rotation.

---

Calculation of radius of gyration of a thin rod

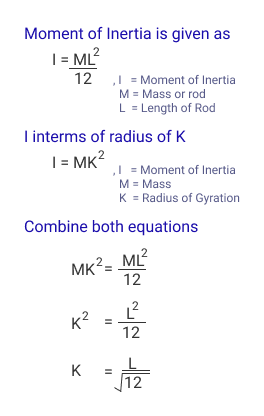
Suppose we have a thin rod of mass M and length L. The moment of inertia of the rod about an axis through the center and perpendicular to its length is

  I = M L2 / 12

From the earlier equation, we know the relation between the moment of inertia and radius of gyration is

  I = MK2

Now, let’s combine the above two equations.

  MK2 = ML2 / 12

  K2  = L2 / 12

  K   = L / √(12)

Hence, if we know the length of the rod, we can calculate the value of K.

---

Calculate the radius of gyration of a solid sphere

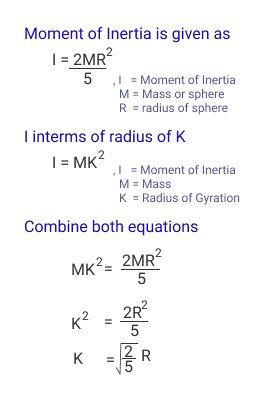
Suppose we have a solid sphere of radius R and mass M. The moment of inertia of the sphere is

  I = 2 MR2 / 5

Since the value of I = MK², the equation becomes

  MK2 = 2 MR2 / 5

Here, K is the radius of gyration.

  K2 = 2 R2 / 5

  K = √(⅖) R

Hence, we can calculate the value of K if we know the radius of the solid sphere.

---

Use of Radius of Gyration

Calculating the radius of gyration is relatively easy, so it is often used to study various dynamic systems. For example,

1. In the molecular system, we can use the radius of gyration (K) to measure the effective size of a polymer. If the value of K is small, then we can say the polymer is relatively compact.

2. Similarly, in structural engineering, we can use K to measure the stiffness of the column.

3. We can also use the radius of gyration to determine the distribution of the mass in the axis of rotation. If the value of K is small, then the mass is close to the axis of rotation. However, if K is large, the mass is far from the axis of rotation.

---

Related Articles

• Coefficient of Friction
• Types of Friction
• Principle of Angular Momentum